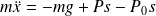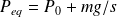Rüchardt's experience
Take 15 minutes to prepare this exercise.
Then, if you lack ideas to begin, look at the given clue and start searching for the solution.
A detailed solution is then proposed to you.
If you have more questions, feel free to ask them on the forum.
Rüchardt's method for determining the ratio
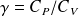of thermal capacity of an ideal gas at constant pressure and volume, studying (see figure) the movement of a ball in a glass tube.
The metal ball, a diameter close to that of the tube behaves as a sealed piston.
Neglecting friction.
When we loose ball in the tube of cross-section
, we observe oscillations around an equilibrium position.
The method consists in measuring the period of oscillation
of the ball in the tube or, equivalently, the period of oscillation of the pressure of the air contained inside the bottle.
For this, pressure is recorded using a pressure sensor for about
.
The air is treated as an ideal gas.
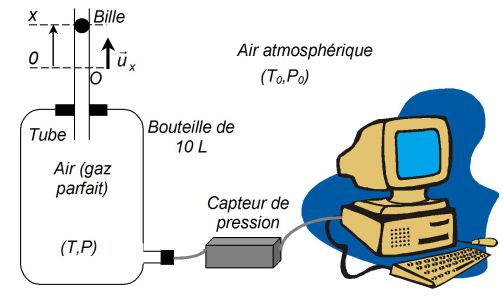
It is noted
the position of the ball center at time
(the origin
is chosen at the equilibrium position of the ball) ;
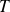and
denote the temperature and pressure of the air inside the bottle.
Data :
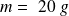(mass of the ball),
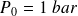and
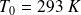(pressure and temperature of atmospheric air),
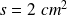(inner cross section of the tube),
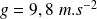(Earth gravity field) and
(total volume, for
).
A Java simulation of this experiment (JJ Rousseau) : click HERE
Question
Applying second law of Newton to the ball, establish the differential equation of motion of the ball.
What is the
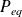pressure balance ?
Question
From a thermodynamic point of view, the compressions and expansions of gas within the bottle are regarded as practically reversible and adiabatic.
The gap of pressure and of volume are low, is approximated
by
and
by
.
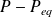Deduce
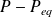as a function of
.
It is assumed that the ball oscillates quickly enough so that air within the bottle does not have time to receive heat transfer from the outside (but still slowly enough that this transformation can be considered reversible !).
Ultimately, the transformation is considered reversible adiabatic (is isentropic).
Therefore, the Laplace law is applicable and is written :
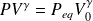
Or, in differential form :
Is here :
Whence :
Question
Determine the differential equation satisfied by
.
What is the period
of oscillations of pressure ?
We measure
.
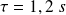Determine the value of
.
Comment the obtained value.
What can be the encountered experimental problems ?
The differential equation of the ball movement then becomes :
Whence :
It is the characteristic differential equation of a harmonic oscillator of angular frequency :
And period :
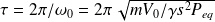
Coefficient
is therefore expressed as a function of the measured period of the pressure oscillations in the bottle :
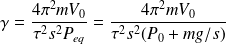
Numerical Application :
We find
.
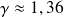The experimental value obtained is consistent with the expected theoretical value (either
) for air which is composed of diatomic molecules (oxygen and nitrous).
The experimental method proposed in this exercise was originally developed by the physicist Rüchardt in
.
This method has limitations because it is based on three assumptions (gas likened to a perfect gas, negligible friction between the ball and the tube of the bottle and reversible adiabatic transformations gas) that are not necessarily verified.
